magnetic movie

https://vimeo.com/1166968

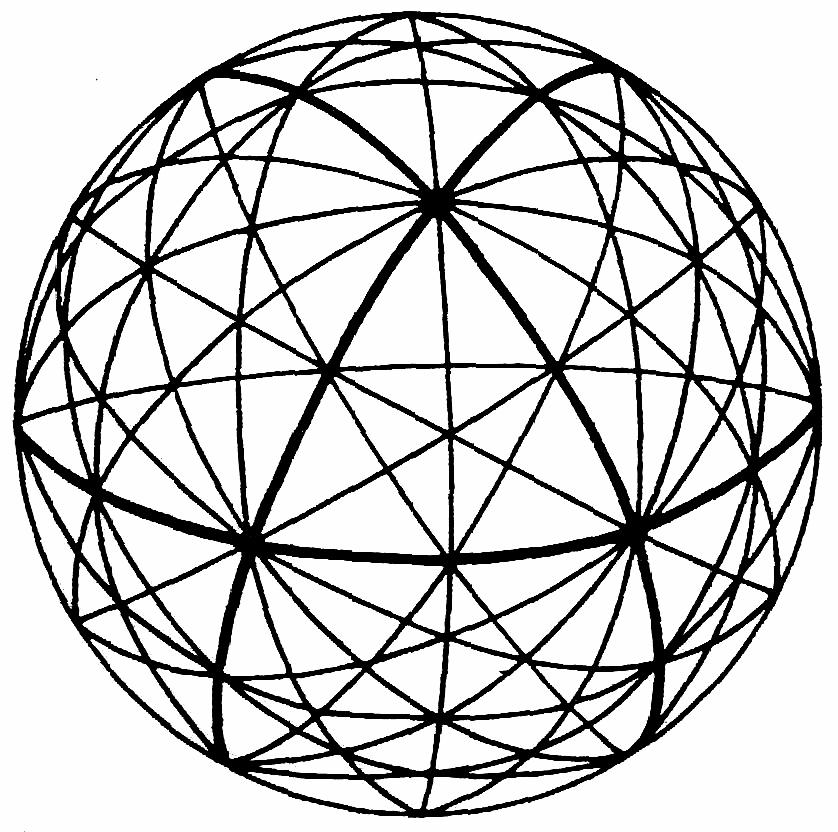
vector equilibrium

1980

Vector Equilibrium and its Transformation Pathways

Comment

- / -

---

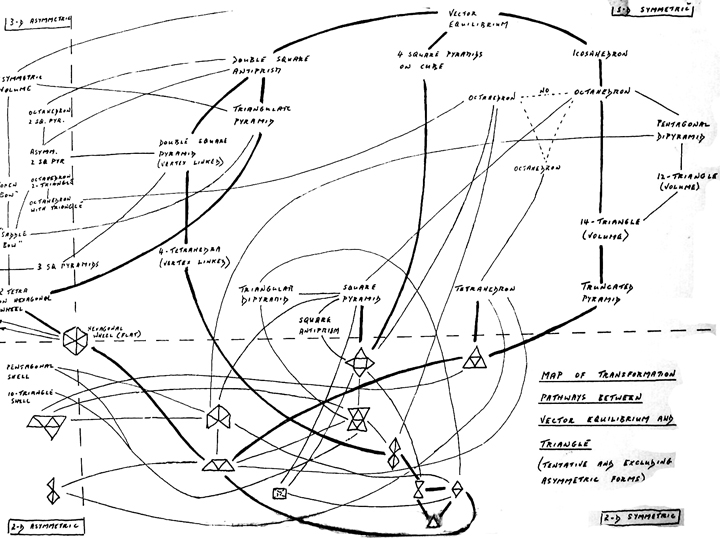
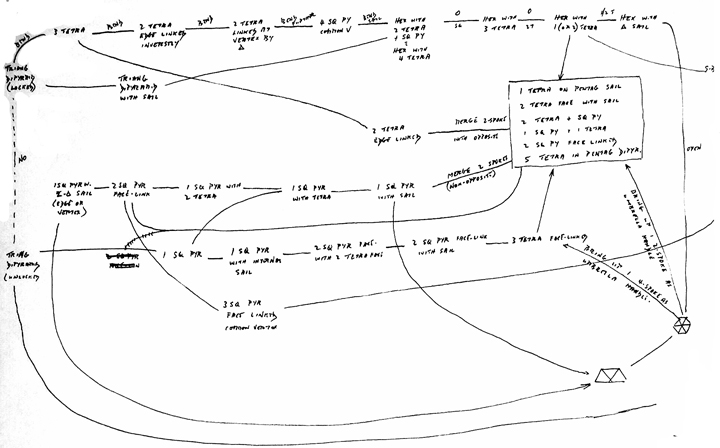
1. Map of the relationships between the forms of the vector equilibrium

The pathways illustrated on the map are the main connections between predominantly symmetric figures which can he derived by subjecting the vector equilbrium (also named a "jitterbug") to various processes: folding, compressing, expanding, rotating, etc

"JITTERBUG" name coined by the American mathematician Buckminster Fuller for the geometrical transform- ations of a flexible jointed cuboctahedron. (R. Buckminster Fuller. Synergetics: Explorations in the Geometry of Thinking MacMillan, 1975)
Omnidirectional closest packing of spheres defining nodes of the Cuboctahedron Vector equilibrium The cuboctahedron is the polyhedron obtained by bisecting the 12 edges and truncating the eight corners of the cube. It can also be developed, however, from the omnidirectional closest packing of spheres around one nuclear sphere. The centres of 12 such spheres define the 12 nodes of the cuboctahedron. As all spheres are the same size it can be seen that the length of the cuboctahedron's edges equal the distance from its centre to its 12 nodes. Thus the form can be considered to be a system of equal vectors which are in equilibrium • a VECTOR EQUILIBRIUM - where the outward radial thrust of the vectors from the centre is balanced by the circumfer- entially restraining chordal vectors. The explosive forces perfectly balance the implosive forces. Because energetic forces are in such an unstable equilibrium, as Buckminster Fuller says,.... "the vector equilibrium is a condition in which nature never allows herself to tarry. The vector equilibrium itself is never found exactly symmetrical in nature's crystallography. Ever pulsive and impulsive, nature never pauses her cycling at equilibrium : she refuses to get caught irrecover- ably at the zero phase of energy. She always closes her transformative cycles at the maximum positive or negative asymmetry stages." The JITTERBUG demonstrates complex geometric relationships in a very simple and entertaining educational device for children and adults. Additional inform- ation is enclosed. Made in Australia. The geometric forms we observe in nature are often those of the JITTERBUG'S transformational patterns such as the icosahedron, the octahedron and the tetrahedron where the enegetic vectors temporarily "fix". The JITTERBUG, then, is a vector equilibrium with the internal radially thrusting vectors removed so that, in manipulating it, the pull of your hands replaces the push of nature, and one can feel nature's energy circuits. HOW TO JITTERBUG With two hands holding opposite triangles and without twisting its axis, the JITTERBUG is symmetrically contracted, firstly to an ICOSAHEDRON and then to an OCTAHEDRON. At this stage, all the struts are doubled together in tight parallel (simulating chemical double bonding of atoms). Now, by rotating the top triangle of the composite octahedron by 60 degrees, and then lowering it, the whole structural - system will transform into a two frequency triangle whose outer triangles can now be folded inwards to form a six edge pattern of the tetrahedron which organic chemists would describe as a quadrivalent tetrahedral structure. Finally, the model of the tetrahedron can be turned inside out, opened up, and the previous contractive sequence of events is reversed. With practice, a rythmic contraction - expansion move- ment can be acquired with alternative clockwise and anti-clockwise rotations. Happy jitterbugging! TETRA TOYS 1 Queens Ave: McMahons Point N.S.W. 2060 Tel: (02)436-1399

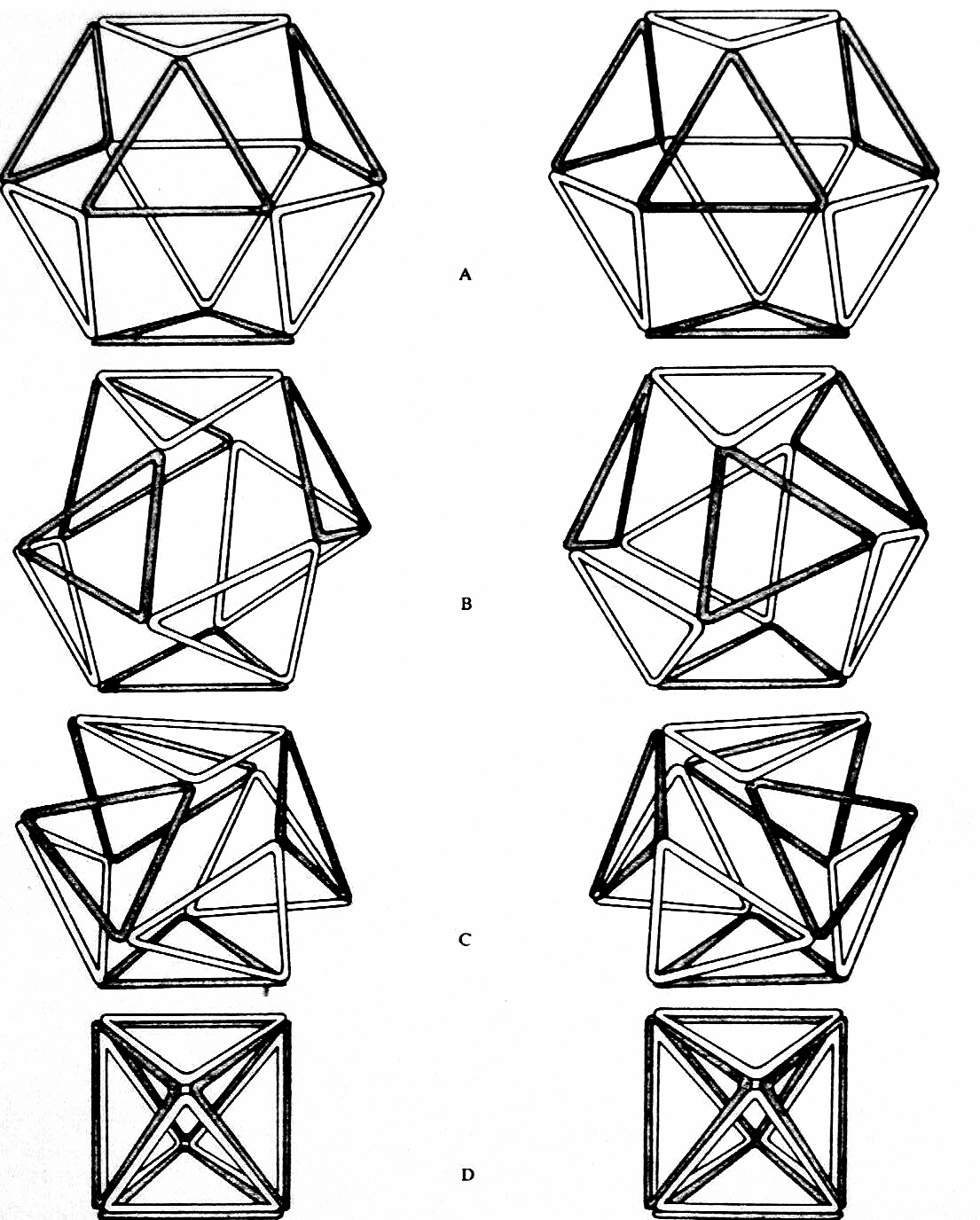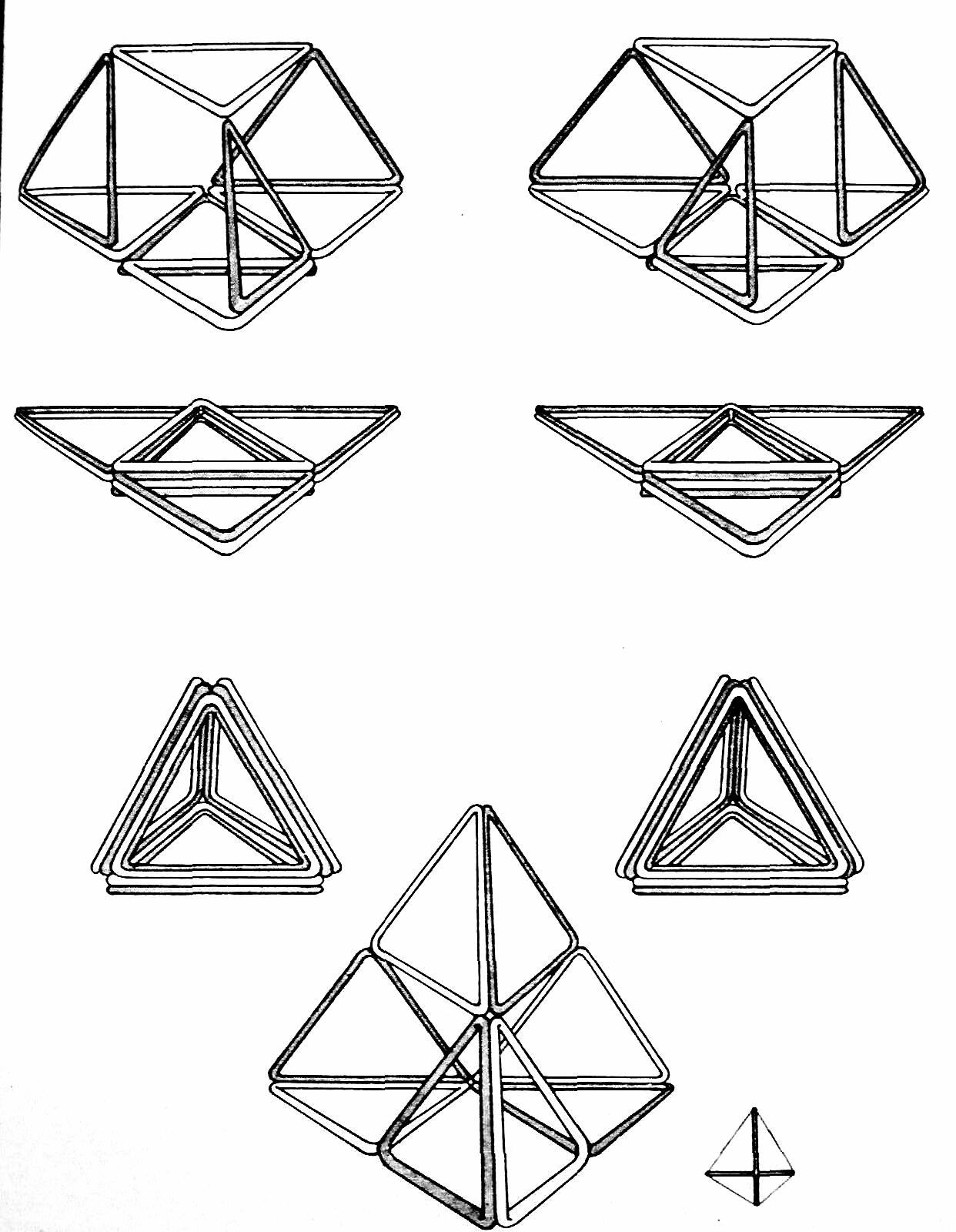The figures emerge whenever a new pattern of triangulation occurs, namely whenever any of the processes brings vertices into triangulation distance, or takes them out of it. Example: in compressing to opposite triangles from the vector equilibrium position, a point is reached when the 6 squares are converted into 12 possible triangles with the (imaginary) addition of six extra edges across the original diagonals

The map is not complete even for the portion shown. The 3-dimensional asymmetric portion is quite complicated and difficult to disentangle. It is interesting that most of those structures should emerge via the hexagonal wheel

Map of transformations of the vector equilibrium by manipulation of the jitterbug

2. Table

The table lists at the top of the page most of the configurations which are indicated on the map. Down the side is a list of numbers. In the squares of the table under each figure are to be found an indication of what aspect of the figure has elements whose total number corresponds to that indicate along the right hand margin. So, for example, against "1", the "square" and the "triangle" each have "F" for "face", because they each have one single face. Against "3", the "triangle" has "V" and "E", because there are 3 vertices and 3 edges to it.

The other codes are as follows:

F = face
A = axis of symmetry
V = vertex (or edge intersection)
E = edge
T = total of F + V ⁺E, namely total "features" to the figure
T2 = T + 2, because a different notion of total would include as extra "features" either:

inside and outside (for a 3-dimensional form) or:
visible and invisible sides (for a 2-dimen.) FV = F + V FE = F + F VE = V + E C = circumferential circles associated with the degree of symmetry

The totals FV, FE, and VE are included because such figures can be used to hang certain concepts, categories, or energies on them and in some cases it is these totals which are significant. Thus a four-fold energy nay be said to manifest through a triangle through FV or VE which total 4 in each case. Note the 32 which emerges in the icosahedron (FV) which also has a 64 (T2).

The table may be used to work out how different forms "carry" different energies at various stages through the transformation pathways on the map

Thus although a 7-fold energy may be carried in "embryonic"form through the total (T) of features. By the time the structure is elaborated into the vector equilibrium form, the 7 is being carried by the 7 axes.

It is interesting to note the transition from "embryonic" representation as just described, through explicit association with edges or faces, to invisible significance as a principle of symmetry as in the case of the axes just mentioned.

It is interesting to note, particularly in the case of the hexagonal wheel, how flat structures must be opened into volumes before such invisible symmetry effects can manifest. In the case of the wheel, retaining a hold on the centre prevents such opening out. The centre has to he released and In the most highly developed forms [vector equilibrium, icosahedron, etc) is defined by the manner in which the visible features are positioned around it. There is then no central vertex.

3. Processes

Some of the accompanying notes describe the transformation processes represented on the map

4. Number details

Other notes describe some of the interesting features associated with the numbers in the table. The table is however more comprehensive in some respects although the notes mention some features not present in the table.

5. Relation to tensegrity

Tensegrities are based on balancing compressive and tensile forces (sticks and strings in models). They have mainly been explored for configurations such as: vector equilibrium (cuboctahedron), icosahedron, octahedron, and tetrahedron.

It is easy to see that it requires two hands [two forces) to maintain the vector equilibrium configuration when it is the sticks [edges) which outline the form. The configuration is thus dependent on "outside" forces. In order to make the structuring forces "internal", extra elements have to he added. Strings could be used to link certain vertices and in this case the strings would be internal to the configuration, although this is not then a "tensegrity"

In the tensegrity, the strings are on the outside and it is they which outline the form. It is the sticks which cross though the inside

So the two forms are intimately related. In the tensegrity everything is balanced but in the stick-only model the person manipulating it is responsible for the form it takes.

6. Collection of printed notes

Some extracts from Buckminster Fuller's book Synergetics are included to give more ideas about the understanding and significance behind the vector equilibrium concept - he has many more pages than are included here

7. My own interest

Very briefly I have written a number of papers exploring the relationship between geometric forms (tensegrities) and the numbers on which they are based and why and how they are chosen. I am interested in the significance for ordering understanding and making new kinds of group organization possible, I see these configurations as very much related to the movement of different types of energy which must be appropriately balanced and transformed. I am particularly interested in their relation to traditional symbol systems based on sets of 3, 4, 5, 7, 9, 12, 14, 22, 32, 52, 64, etc numbers

I find it highly significant that this "toy" [for I bought it in a toy shop for infants in Australia) should make explicit how to transform between different configurations characterized by different numbers.

I hope that you will enjoy exploring its possibilities from your own point of view. Any comments would be appreciated. I hope to order my own thoughts more coherently over the next few weeks.

Summary of processes indicated on the transformation map

PROCESS I

1. Vector equilibrium
2. Icosahedron	compression of any 2 parallel triangles; triangulation of 6 squares
3. Octahedron	compression completed; elimination of 6 original squares
4. "14-Triangle form"	rotation of one triangle with respect to its parallel; open up 3 squares and triangulate
5. Truncated triangular pyramid	continue rotation; open 3 squares further hut triangulate opposite corners
6. Flat 4-Triangle (a triangle)	rotation completed; squares eliminated
7. Flat 3-Triangle (trapezium)	fold up one triangle
8. Flat 2-Triangle (diamond)	fold up second triangle
9. Flat 1-Triangle (triangle)	fold up third triangle

This process may of course be reversed. Note at the vector equilibrium point that compression can twist the structure in a right or left-handed direction. Both lead through the various steps above down to the triangle. At this point the triangle may be unfolded the other way around, namely effectively turning the whole structure inside-out.

PROCESS II

1. Vector equilibrium
2. "Double square antiprism"	decompressing any of 2 parallel squares; triangulation of the 4 other squares
3. Double square pyramid (linked at vertex)	decompression continued; elimination of the 4 squares
3a Double square pyramid. partially linked at vertex and with 4 tetrahedra	Optional intermediary step: pressure applied to only two opposing vertices; the other two expan outwards creating two triangulation zones
4. "4-Tetrehedra in 2 groups linked at common vertex"	decompression continued; triangulation of 2 remaining squares
4a "2-Tetrahedra on vertexof square pyramid"	Assymetric option: triangulation of 1 square only
4b "2-Triangle sail on vertex of square pyramid"	Assymetric option: elimination of 1 square only
4c "2-Triangle sail on vertex of 2-Tetrahedra"	Assymetric option: elimination of 1 square; triangulation of the other
5. Flat 4-Triangle (double	decompression completed; elimination of all diamond) squares
6. Flat 2-Triangle (vertex link)	fold over on common axis
6a. (diamond)	fold over on common vertex
6b Flat 3-Triangle	fold over of only one triangle
7. Flat 1-Triangle	fold over

PROCESS III

1. Vector equilibrium
2. "4-Square pyramids on cube"	compression on any of 2 parallel square faces; triangulation of 4 other squares
3. Plat 4 triangles on square	compression completed; elimination of 4 original squares
4.	Fold over one triangle
5.	fold over second triangle
6.	fold over third triangle
7. Flat square(to 9)	fold over fourth triangle
7a Square pyramid	when folding is to a common vertex
8. Triangular dipyramid
9. Flat diamond
10.Triangle

PROCESS IV

1. Vector equilibrium
2. "4-Square pyramids on cube"	compression of any of 2 parallel square faces; triangulation of 4 other squares
3. Flat 4 triangles on square	compression completed; elimination of 4 original squares
4. Flat 6-triangle	compression of 2 opposing inner vertices and triangulation of that square
5. Flat 4-triangle (linked diamonds)	compression completed; elimination of square
6. Flat 2-triangle (vertex link)	fold over common axis
6a Flat 2-triangle (diamond)	fold over common vertex
6b Flat 3-triangle	fold over of only one triangle
7. Flat 1-triangle	fold over

PROCESS V

1. Vector equilibrium
2. Icosahedron
3. Octahedron
4. Pentagonal dipyramid (10 triangle)	sliding open and triangulating one square
5. 12-triangle form	sliding open and triangulating second square
6. 14-triangle form	sliding open and triangulating third square
7. 16-Triangle/2-Square form	sliding open and triangulating fourth square
3. Vector equilibrium	opening all squares; eliminating triangulation

Number details

(mainly incorporated into the table)

1: ***

1. The structure remains coherent in what ever way it is twisted and in whatever configuration it takes
2. Some configurations are more elegantly symmetrical and reveal this unity more clearly
3. This unity acquires special significance when the structure is in any of the classical three-dimensional configurations: tetrahedron, octahedron, icosahedron (known as the Platonic solids), or vector equilibrium (an Archimedian solid) also known as cuboctahedron
4. Vector equilibrium: can be folded up to a single triangle configuration beyond which it can be reduced no further by "conventional" manipulation
5. In contrast to many other configurations, a few can be kept with the application of force at only one point. These include: tetrahedron, square pyramid, double square pyramid (force at common vertex),double tetrahedron (force at common vertex)

2: ***

1. The fundamental two-ness of the structure is evident from the parallelism between paris of edges in many of the more symmetrical configurations, particularly in three-dimensions
2. The edges are themselves basic two-dimensional constructional elements necessary to bring any form into manifestation
3. The importance of two-ness is also illustrated by the opposing forces (e.g. two hands) required in order to maintain many of the three-dimensional configurations and reveal their symmetry
4. Many of the configurations can be seen as made up of two equal halves due to their bilateral symmetry
5. The flat configurations have two sides
6. The three-dimensional configurations may have an inside and an outside (others are intersecting flat planes)

3: ***

1. The structure is made up of 8 triangles. It is a demonstration of possible triangular configurations and of ways in which the triangle can be "opened up" or developed
2. Vector equilibrium: The squares are in facing parallel pairs. The common axis through the centre of the squares of each pair results in 3 such axes meeting at right angles at the centre of the vector equilibrium
3. Octahedron: There are 3 circuits of edges around the circumference of the form ("great circles"). The planes of the circuits meet at right angles at the centre
4. Triangle: In the falt one-triangle configuration, there are 3 vertices and 3 edges
5. 3-Triangle: In its flat three-triangle configuration, there are 3 faces

4: ***

1. Vector equilibrium:
   - The 6 squares ore one manifestation of four-ness
   - The common axis through the centres of the triangles (B in four parallel pairs) results in 4 such axes meeting at right angles at the centre of the vector equilibrium
   There are 4 circuits of edges around the circumference (great circles), of which 3 delineate each triangle and one constitutes a parallel plane to it
2. Icosahedron: There are 4 groups of 5-triangle pentagonal groups, making the total of 20 faces
3. Octahedron: The B triangular faces are in parallel pairs. The common axis through the centre of the triangles ofreach pair results in 4 such axes meeting at right angles at the centre of the octahedron
   - There are 4 edges in each of the 3 circuits of edges around the circumference
4. 4-Triangle: In its flat four-triangle configuration, there ane 4 faces
5. 2-Triangle: In its flat two-triangle configuration, there are 4 vertices
6. 1-Triangle: In its one-triangle configuration, the single face and the 3 edges constitute 4 features, as do the single face and the 3 vertices

5: ***

1. Vector equilibrium:Riven the presence of a 13th sphere (see point 13 below), each of the 12 outer spheres touches 4 others plus a 5th, namely the 13th at the centre
2. Icosahedron: Each square of the vector equilibrium is effectively eliminated by the addition of one extra edge (triangulating the square). As a result 5 edges (one of them imaginary here) meet at each vertex
   - Each vertex is surrounded by 5 triangles whose bases constitute a pentagonal "lesser" circuit (of which there are 12)
   - There are 5 greater circuits around the circumference, each with B edges delineating hexagons whose planes all intersect at the centre point
3. 2-Triangle: In the flat two-triangle configuration there are 5 edges
4. 3-Triangle: In the flat three-tirnagle configuration there are 5 vertices

6: ***

1. Vector equilibrium: There are 6 squares in parallel pairs. The axis through the centres of the squares of each pair meet at the centre at right angles.
   - The lines from each vertex to the centre make of them 6 square pyramids
2. Icosahedron: There are 5 "great" circuits of edges around the circumference, each with 6 edges delineating hexagons whose planes all intersect at the centre point
   - In contracting from the vector equilibrium configuration, 6 extra edges are required to maintain the icosahedron by triangulating the original squares
3. Octahedron: The edges meet at B vertices
4. Tetrahedron: There are 6 edges
5. 4-Triangle: In the flat four-triangle configuration there are 6 vertices
6. 2-Triangle: In the flat two-triangle configuration, the 2 faces and 4 vertices constitute B features
7. 1-Triangle: In the flat one-triangle configuration, the 3 edges and 3 vertices constitute 6 features

7: ***

1. Vector equilibrium: The axes through the centre of the parallel faces result in ' 3 axes from the square faces and 4 axes from the triangular faces. All axes intersect at the centre of the vector equilibrium
2. Pentagonal dipyramid: Sliding one triangle in relation to its parallel triangle inanoctahedron configuration results in a pentagonal dipyramid with 7 vertices
3. 3-Trlangle: In the flat three-triangle configuration, there are 7 edges
4. 2-Triangle: In the flat two-triangle configuration, the 2 faces and 5 edges constitute 7 features
5. 1-Triangle: In the flat one-triangle configuration, the single face, 3 edges and 3 vertices constitute 7 features

8: ***

1. Vector equilibrium: There are 8 triangular faces in parallel pairs.
   - The lines linking each vertex to the centre make of them 8 tetrahedrons
2. Octahedron: There are 8 triangular faces in parallel pairs
   - The lines linking each vertex to the centre make of them 8 tetrahedra
3. Tetrahedron: The 4 vertices and 4 faces constitute 8 features
4. 3-Triangle: In its flat three-triangle configuration, the 3 faces and 5 vertices constitute 8 features

Some other numbers

13: ***

1. Vector equilibrium: Spheres of eaqual radius centred on each vertex touch four other neighbouring spheres and create a space for a 13th sphere of the same radius at the centre. This 13th sphere centred on an invisible vertex touches ell 12 outer spheres, which are thus each in contact with 5 spheres [4 outer and 1 inner)
2. Icosahedron: The contraction from the vector equilibrium is that which would result from the removal of a 13th central sphere.
3. Hexagonal wheel: The total of 6 faces and 7 vertices makes 13 features. The central visible vertex is the 13th feature

22: ***

1. Pentagonal dipyramid: The total of 7 vertices and 15 edges makes 22 features.

32: ***

1. a. Pentagonal dipyramid: The total of 7 vertices, 15 edges and 10 faces makes 32 features
2. b. Icosahedron: The total of 20 faces and 12 vertices makes 32 features
3. c. Vector equilibrium: The 8 facial triangles may be considered as the bases of B tetrahedra with their vertices at the centre. The total number of faces of these tetrahedra is 32

52: ***

1. a.Vector equilibrium: The 14 faces, 12 vertices and 24 edges make a total of 50 features, to which may be added 2 for the inside and the outside, making 52 features

64: ***

1. a. Icosahedron: The 20 faces, 12 vertices and 30 edges make a total of 62 features, to which may be added 2 for the inside and the outside, making 64

Collection of notes on the vector equilibirum Extracts from: R Buckminster Fuller (Synergetics: explorations in the geometry of thinking. Macmillan, 1975 876 pages) (Second volume also)

430.02 It is called the vector equilibrium because the radials and the cir- cumferentials arc all of the same dimension and the tendencies to both ex- plode and implode are symmetrical. That the explosive and implosive forces are equal is shown by the four-dimensional hexagonal cross sections whose radial and circumferential vectors balance. The eight triangular faces reveal four opposite pairs of single-bonded tetrahedra in a positive and negative tetrahedral system array with a common central vertex and with coinciding ra- dial edges. The four hexagonal planes (hat cross each other at the center of the vector-equilibrium system are parallel to the four faces of each of its eight tetrahedra. Six square faces occur where the six half-octahedra converge around the common vector-equilibrium nuclear vertex. 430.03 In terms of vectorial dynamics, the outward radial thrust of the vector equilibrium is exactly balanced by the circumferentially restraining chordal forces: hence the figure is an equilibrium of vectors. All the edges of the figure are of equal length, and this length is always the same as the dis- tance of any of its vertexes from the center of the figure. The lines of force radiating from its center are restrainingly contained by those binding inward arrayed in finite closure circumferentially around its periphery-barrel-hoop- ing. The vector equilibrium is an omnidirectional equilibrium of forces in which the magnitude of its explosive potentials is exactly matched by the strength of its external cohering bonds. If its forces are reversed, the magni- tude of its contractive shrinkage is exactly matched by its external compres- sive archwork's refusal to shrink. 430.04 The vector equilibrium is a truncated cube made by bisecting the edges and truncating the eight corners of the cube to make the four axes of the four planes of the vector equilibrium. The vector equilibrium has been called the "cuboctahedron" or "cubo-octahedron" by crystallographers and geo- meters of the non-experimentally-informed and non-cnergy-concerned past. As such, it was one of the original 23 Archimedean "solids," 430.05 The vector equilibrium is the common denominator of the tetrahe- dron, octahedron, and cube. It is the decimal unit within the octave system. Double its radius for octave expansion. 430.06 The vector equilibrium is a system. It is not a structure. Nor is it a prime volume, because it has a nucleus. It is the prime nucleated system. The eight tetrahedra and the six half-octahedra into which the vector equilibrium may be vectorially subdivided are the volumes that are relevantly involved. 440.00 Vector Equilibrium as Zero Model 440.01Equilibrium between positive and negative is zero. The vector equilibrium is the true zero reference of the energetic mathematics. Zero pul- sation in the vector equilibrium is the nearest approach we will ever know to eternity and god: the zerophase of conceptual integrity inherent in the positive and negative asymmetries that propagate the differentials of consciousness. 440.02 The vector equilibrium is of the greatest importance to all of us because all the nuclear tendencies to implosion and explosion are reversible and are always in exact balance. The radials and the circumferentials are in balance. But the important thing is that the radials, which would tend to explode since they are outwardly pushing, are always frustrated by the tensile finiteness of the circumferential vectors, which close together in an orderly manner to cohere the disorderly asundering. When the radial vectors are tensilely contractive and separately implosive, they are always prevented from doing so by the finitely closing pushers or compressors of the circumferential set of vectors. The integrity of Universe is implicit in the external finiteness of the circumferential set and its surface-layer, close-packing, radius-contracting proclivity which always encloses the otherwise divisive internal radial set of omnidirectional vectors. 440.03 All the internal, or nuclear, affairs of the atom occur internally to the vector equilibrium. All the external, or chemical, compoundings or asso- ciations occur externally to the vector equilibrium. All the phenomena exter- nal to-and more complex than-the five-frequency vector equilibria relate to chemical compounds. Anything internal to-or less complex than-the five- frequency vector equilibrium relates principally to single atoms. Single atoms maintain omnisymmetries; whereas chemical compounds may associate as polarized and asymmetrical chain systems. 440.04 The vector equilibrium is the anywhere, anywhen, eternally re- generative, event inceptioning and evolutionary accommodation and will never be seen by man in any physical experience. Yet it is the frame of evolvement. It is not in rotation. It is sizeless and timeless. We have its math- ematics, which deals discretely with the chordal lengths. The radial vectors and circumferential vectors are the same size. 440.05 The vector equilibrium is a condition in which nature never allows herself to tarry. The vector equilibrium itself is never found exactly symmetri- cal in nature's crystallography. Ever pulsive and impulsive, nature never pauses her cycling at equilibrium: she refuses to get caught irrecoverably at the zero phase of energy. She always closes her transformative cycles at the maximum positive or negative asymmetry stages. See the delicate crystal asymmetry in nature. We have vector equilibriums mildly distorted to asym- metry limits as nature pulsates positively and negatively in respect to equilib- rium. Everything that we know as reality has to be either a positive or a nega- tive aspect of the omnipulsative physical Universe. Therefore, there will always be positive and negative sets that are ever interchangeably intertrans- formative with uniquely differentiable characteristics. 440.06 The vector equilibrium is at once the concentric push-pull in- terchange, vectorial phase or zone, of neutral resonance which occurs be- tween outwardly pushing wave propagation and inwardly pulling gravitational coherence. 440.07 All the fundamental forms of the crystals are involved in the vec- tor equilibrium. It is a starting-point-not anything in its own right-if it is a vector equilibrium. 440.08 As the circumferentially united and finite great-circle chord vec- tors of the vector equilibrium cohere the radial vectors, so also does the meta- physical cohere the physical. 441.00 Vector Equilibrium as Zero Tetrahedron 441.01Emptiness at the Center: All four planes of all eight tetrahe- dra, i.e., 32 planes in all, are congruent in the four visible planes passing through their common vector equilibrium center. Yet you see only four planes. Both the positive and the negative phase of the tetrahedra are in congruence in the center. They are able to do this because they are synchro- nously discontinuous. Their common center provides the locale of an abso- lutely empty event. 441.02 Vector equilibrium accommodates all the intertransformings of any one tetrahedron by polar pumping, or turning itself inside out. Each vec- tor equilibrium has four directions in which it could turn inside out. It uses all four of them through the vector equilibrium's common center and generates eight tetrahedra. The vector equilibrium is a tetrahedron exploding itself, turn- ing itself inside out in four possible directions. So we get eight: inside and outside in four directions. The vector equilibrium is all eight of the potentials. 443.00 Vector Equilibrium as Equanimity Model 443.01 In order to reduce the concept of vector equilibrium to a single- name identity, we employ the word equanimity as identifying the eternal metaphysical conceptuality model that eternally tolerates and accommodates all the physically regenerative intertransforming transactions of eternal, inex- orable, and irreversible evolution's complex complementations, which are unitarily unthinkable, though finite. 443.02 The equanimity model permits metaphysically conceptual think- ability and permits man to employ the package-word Universe. Equanimity, the epistemological model, is the omni-intertransformative, angle- and frequency-modulatable, differential accommodator and identifies the direction toward the absolute, completely exquisite limit of zero-error, zero-time om- nicomprehension toward which our oscillatory, pulsating reduction of tol- erated cerebrally reflexed aberrations trends. 443.03 Humanity's physical brains' inherent subjective-to-objective time lag reflexing induces the relatively aberrated observation and asymmetrical ar- ticulation tolerated by ever more inclusively and incisively demanding mind's consciousness of the absolute exactitude of the eternally referential centrality at zero of the equanimity model. Thus mind induces human consciousness of evolutionary participation to seek cosmic zero. Cosmic zero is conceptually but sizelessly complex, though full-size-range accommodating. 443.04 In the equanimity model, the physical and the metaphysical share the same design. The whole of physical Universe experience is a consequence of our not seeing instantly, which introduces time. As a result of the gamut of relative recall time-lags, the physical is always the imperfect experience, but tantalizingly always ratio-equated with the innate eternal sense of perfection. 451.00 Vector Equilibrium: Axes of Symmetry and Points of Tangency in Closest Packing of Spheres 451.01 It is a characteristic ofall the 25 great circles that each one of them goes through two or more of the vector equilibrium's 12 vertexes. Four of the great circles go through six vertexes; three of them go through four ver- texes; and 18 of them go through two vertexes. 451.02 We find that all the sets of the great circles that can be generated by all the axes of symmetry of the vector equilibrium go through the 12 ver- texes, which coincidentally constitute the only points of tangency of closest- packed, uniform-radius spheres. In omnidirectional closest packing, we always have 12 balls around one. The volumetric centers of the 12 uniform- radius balls closest packed around one nuclear ball are congruent with the 12 452.03 Great Circles of Vector Equilibrium 169 vertexes of the vector equilibrium of twice the radius of the closest-packed spheres. 451.03 The network of vectorial lines most economically interconnecting the volumetric centers of 12 spheres closest packed around one nuclear sphere of the same radius describes not only the 24 external chords and 12 radii of the vector equilibrium but further outward extensions of the system by closest packing of additional uniform-radius spheres omnisurrounding the 12 spheres already closest packed around one sphere and most economically intercon- necting each sphere with its 12 closest-packed tangential neighbors, altogether providing an isotropic vector matrix, i.e., an omnidirectional complex of vec- torial lines all of the same length and all interconnected at identically angled convergences. Such an isotropic vector matrix is comprised internally entirely of triangular-faced, congruent, equiedged, equiangled octahedraand telra- hedra. This isotropic matrix constitues the omnidirectional grid. 451.04 The basic gridding employed by nature is the most economical agglomeration of the atoms of any one element. We find nature time and again using this closest packing for most economical energy coordinations. 452.00 Vector Equilibrium: Great-Circle Railroad Tracks of Energy 452.01 The 12 points of tangency of unit-radius spheres in closest pack- ing, such as is employed by any given chemical element, are important be- cause energies traveling over the surface of spheres must follow the most eco- nomical spherical surface routes, which are inherently great circle routes, and in order to travel over a series of spheres, they could pass from one sphere to another only at the 12 points of tangency of any one sphere with its closest- packed neighboring uniform-radius sphere. 452.02 The vector equilibrium's 25 great circles, all of which pass through the 12 vertexes, represent the only "most economical lines" of en- ergy travel from one sphere to another. The 25 great circles constitute all the possible "most economical railroad tracks" of energy travel from one atom to another of the same chemical elements. Energy can and does travel from sphere to sphere of closest-packed sphere agglomerations only by following the 25 surface great circles of the vector equilibrium, alwas accomplishing the most economical travel distances through the only 12 points of closest- packed tangency. Fig. 450.11 A Axes of Rotation of Vector Eguilibrium: A. Rotation of vector equilibrium on axes through centers of opposite triangular faces defines four equatorial great-circle planes. B. Rotation of the vector equilibrium on axes through centers of opposite square laces defines three equatorial great-circle planes. C. Rotation of vector equilibrium on axes through opposite vertexes defines six equa- torial great-circle planes. D. Rotation of the vector equilibrium defining twelve equatorial great-circle planes, each of which passes through two opposite vertexes and two midpoints of the edges of two opposite triangular faces. The axes of rotation pass through opposite square faces. Fig. 450.11BProjection of 25 Great-Circle Planes in Vector Equilibrium System: The complete vector equilibrium system of 25 great-circle planes, shown as both a plane faced-figure and as the complete sphere (3 + 4 + 6 + 12 = 25). The heavy lines show the edges, of the original 14-faced vector equilibrium. Fig. 457.30A Axes of Rotation of Icosahedron: A. The rotation of the icosahedron on axes through midpoints of opposite edges define 15 great-circle planes. B. The rotation of the icosahedron on axes through opposite vertexes define six equatorial great-circle planes, none of which pass through any vertexes. C. The rotation of the icosahedron on axes through the centers of opposite faces define ten equatorial great-circle planes, which do not pass through any vertexes. Fig. 457.30B Projection of 31 Great-Circle Planes in Icosahedron System; The com- plete icosahedron system of 31 great-circle planes shown with the planar icosahedron as well as true circles on a sphere (6 + 10 + 15 = 31). The heavy lines show the edges of the original 20-faced icosahedron. Fie. 457.40 Definition of Spherical Polyhedral in 31 -Great-Circle Icosahedron System: The 31 great circles of the spherical icosahedron provide spherical edges for three other polyhedra in addition to the icosahedron itself, whose edges are shown as heavy lines. The shading indicates a typical face, as follows: A. The rhombic triacontrahedron with 30 spherical rhombic faces, each consisting of four basic, least-common-denominalor triangle. B. The octahedron with 15 basic, least-conimon-denominalor spherical triangles. C. The pentagonal dodecahedron with ten basic. least-common-denominator spherical triangles. 458.04 Great Circles of Icosahedron 187 457.43 The spherical pentagonal dodecahedron is composed of 12 spheri- cal pentagons. 458.00 Icosahedron: Great Circle Railroad Tracks of Energy 458.01Whereas each of the 25 great circles of the vector equilibrium and the icosahedron goes through the 12 vertexes at least twice; and whereas the 12 vertexes are the only points of intertangency of symmetric, unit-radius spheres, one with the other, in closest packing of spheres; and inasmuch as we find that energy charges always follow the convex surfaces of systems; and inasmuch as the great circles represent the most economical, the shortest distance between points on spheres; and inasmuch as we find that energy always takes the most economical route; therefore, it is perfectly clear that energy charges passing through an aggregate of closest-packed spheres, from one to another, could and would employ only the 25 great circles as the great- circle railroad tracks between the points of tangency of the spheres, ergo, be- tween points in Universe. We can say, then, that the 25 great circles of the vector equilibrium represent all the possible railroad tracks of shortest energy travel through closest-packed spheres or atoms. 458.02 When the nucleus of the vector equilibrium is collapsed, or con- tracted, permitting the 12 vertexes to take the icosahedral conformation, the 12 points of contact of the system go out of register so that the 12 vertexes that accommodate the 25 great circles of the icosahedron no longer constitute the shortest routes of travel of the energy. 458.03 The icosahedron could not occur with a nucleus. The icosahedron, in fact, can only occur as a single shell of 12 vertexes remote from the vector equilibrium's multi-unlimited-frequency, concentric-layer growth. Though it has the 25 great circles, the icosahedron no longer represents the travel of energy from any sphere to any tangent sphere, but it provides the most eco- nomical route between a chain of tangent icosahedra and a face-bonded icosa- hedral structuring of a "giant octahedron's" three great circles, as well as for energies locked up on its surface to continue to make orbits of their own in local travel around that single sphere's surface. 458.04 This unique behavior may relate to the fact that the volume of the icosuhedron in respect to the vector equilibrium with the rational value of 20 is 18.51 and to the fact that the mass of the electron is approximately one over 18.51 in respect to the mass of the neutron. The icosahedron's shunting of energy into local spherical orbiting, disconnecting it from the closest-packed railroad tracks of energy travel from sphere to sphere, tends to identify the icosahedron very uniquely with the electron's unique behavior in respect to nuclei as operating in remote orbit shells 460.00 Jitterbug: Symmetrical Contraction of Vector Equilibrium 460.01 Definition 460.011The "jitterbug" is the finitely closed, external vector structuring of a vector-equilibrium model constructed with 24 struts, each representing the push-pull, action-and-reaction, local compression vectors, all of them cohered tensionally to one another's ends by flexible joints that carry only ten- sion across themselves, so that the whole system of only-locally-effective compression vectors is comprehensively cohered by omniembracing continu- ous four closed hexagonal cycles' tension. 460.02 When the vector-equilibrium "jitterbug" assembly of eight trian- gles and six squares is opened, it may be hand-held in the omnisymmetry con- formation of the vector equilibrium "idealized nothingness of absolute mid- dleness." If one of the vector equilibrium's triangles is held by both hands in the following manner-with that triangle horizontal and parallel to and above a tabletop; with one of its apexes pointed away from the holder and the bal- ance of the jitterbug system dangling symmetrically; with the opposite and lowest triangle, opposite to the one held, just parallel to and contacting the tabletop, with one of its apexes pointed toward the individual who is hand- holding the jitterbug-and then the top triangle is deliberately lowered toward the triangle resting on the table without allowing either the triangle on the table or the triangle in the operator's hands to rotate (keeping hands clear of the rest of the system), the whole vector equilibrium array will be seen to be both rotating equatorially, parallel to the table but not rotating its polar-axis triangles, the top one of which the operating individual is hand-lowering, while carefully avoiding any horizontal rotation of, the top triangle in respect to which its opposite triangle, resting frictionally on the table, is also neither rotating horizontally nor moving in any direction at all. 460.03 While the equatorial rotating results from the top triangle's rota- tionless lowering, it will also be seen that the whole vector-equilibrium array is contracting symmetrically, that is, all of its 12 symmetrically radiated ver- texes move synchronously and symmetrically toward the common volumetric- center of the spherically chorded vector equilibrium. As it contracts compre- hensively and always symmetrically, it goes through a series of geometrical- transformation stages. It becomes first an icosahedron and then an octahedron with all of its vertexes approaching one another symmetrically and without twisting its axis. 460.04 At the octahedron stage of omnisymmetrical contraction, all the vectors (strut edges) are doubled together in tight parallel, with the vector equilibrium's 24 struts now producing two 12-strut-edged octahedra congruent with one another. If the top triangle of the composite octahedron (which is the triangle hand-held from the start, which had never been rotated, but only lowered with each of its three vertexes approaching exactly perpendicularly toward the table) is now rotated 60 degrees and lowered further, the whole structural system will transform swiftly into a tetrahedron with its original 24 edges now quadrupled together in the six-edge pattern of the tetrahedron, with four tetrahedra now congruent with one another. Organic chemists would describe it as a quadrivalent tetrahedral structure. 460.05 Finally, the model of the tetrahedron turns itself inside out and os- cillates between inside and outside phases. It does this as three of its four tri- angular faces hinge open around its base triangle like a flower bud's petals opening and hinging beyond the horizontal plane closing the tetrahedron bud below the base triangle. 460.06 As the tetrahedron is opened again to the horizontal four-triangle condition, the central top triangle may again be lifted, and the whole contrac- tive sequence of events from vector equilibrium to tetrahedron is reversed; the system expands after attaining the octahedral stage. When lifting of the top- held, nonhorizontally rotated triangle has resulted in the whole system ex- panding to the vector equilibrium, the equatorial rotational momentum will be seen to carry the rotation beyond dead-center, and the system starts to contract itself again. If the operating individual accommodates this momentum trend and again lowers the top triangle without rotating it horizontally, the rotation will reverse its original direction and the system will contract through its previous stages but with a new mix of doubled-up struts. As the lowering and raising of the top triangle is continuously in synchronization with the rotating- contracting-expanding, the rotation changes at the vector equilibrium's "zero"-this occasions the name jitterbug. The vector equilibrium has four axial pairs of its eight triangular faces, and at each pair, there are different mixes of the same struts. 460.07 The jitterbug employs only the external vectors of the vector equi- librium and not its 12 internal radii. They were removed as a consequence of observing the structural stability of 12 spheres closest packed around a nuclear sphere. When the nuclear sphere is removed or mildly contracted, the 12 balls rearrange themselves (always retaining their symmetry) in the form of the icosahedron. Removal of the radial vectors permitted contraction of the model-and its own omnisymmetrical pulsation when the lowering and raising patterns are swiftly repeated. It will be seen that the squares accommodate the jitterbug contractions by transforming first into two equiangular triangles and then disappearing altogether. The triangles do not change through the transformation in size or angularity. The original eight triangles of the vector equilibrium are those of the octahedron stage, and they double together to form the four faces of the tetrahedron. 460.08 In the jitterbug, we have a sizeless, nuclear, omnidirectionally pulsing model. The vector-equilibrium jitterbug is a conceptual system in- dependent of size, ergo cosmically generalizable. (See Sees. 515.10 and 515.11.) 461.00 Recapitulation: Polyhedral Progression in Jitterbug 461.01 If the vector equilibrium is constructed with circumferential vec- tors only and joined with flexible connectors, it will contract symmetrically, due to the instability of the square faces. This contraction is identical to the contraction of the concentric sphere packing when the nuclear sphere is re- moved. The squares behave as any four balls will do in a plane. They would like to rest and become a diamond, to get into two triangles. They took up more room as a square, and closer packing calls for a diamond. The 12 ver- texes of the vector equilibrium simply rotate and compact a little. The center ball was keeping them from closer packing, so there is a little more compac- tibility when the center ball goes out. 461.02 Icosahedron: The icosahedron occurs when the square faces are no longer squares but have become diamonds. The diagonal of the square is considerably longer than its edges. But as we rotate the ridge pole, the dia- monds become the same length as the edge of the square (or, the same length as the edge of the tetrahedron or the edge of the octahedron). It becomes the octahedron when all 30 edges are the same length. There are no more squares. We have a condition of omnitriangulation. 461.03 We discover that an icosahedron is the first degree of contraction of the vector equilibrium. We never catch the vector equilibrium in its true existence in reality: it is always going one way or the other. When we go to the icosahedron, we get to great realities. In the icosahedron, we get to a very prominent fiveness: around every vertex you can always count five. 461.04 The icosahedron contracts to a radius less than the radii of the vector equilibrium from which it derived. There is a sphere that is tangent to the other 12 spheres at the center of an icosahedron, but that sphere is in- herently smaller. Its radius is less than the spheres in tangency which generate the 12 vertexes of the vector equilibrium or icosahedron. Since it is no longer Fig. 460.08 Symmetrical Contraction of Vector Equilibrium: Jitterbug System: If the vector equilibrium is constructed with circumferential vectors only and joined with flexible connectors, it will contract symmetrically due to the instability of the square faces. This contraction is identical to the contraction of the concentric sphere packing when its nuclear sphere is removed. This system of transformation has been referred to as the "jitterbug." Various phases are shown in both left- and right-hand contraction: A. Vector equilibrium phase: the beginning of the transformation. B. Icosahedron phase: When the short diagonal dimension of the quadrilateral face is equal to the vector equilibrium edge length. 20 equilateral triangular faces are formed. C. Further contraction toward the octahedron phase. D. Octahedron phase: Note the doubling of the edges. **** 194 System 461.04 the same-size sphere, it is not in the same frequency or in the same energetic dimensioning. The two structures are so intimate, but they do not have the same amount of energy. For instance, in relation to the tetrahedron as unity, the volume of the icosahedron is 18.51 in respect to the vector equilibrium's volume of 20. The ratio is tantalizing because the mass of the electron in re- spect to the mass of the neutron is one over 18.51. That there should be such an important kind of seemingly irrational number provides a strong contrast to all the other rational data of the tetrahedron as unity, the octahedron as four, the vector equilibrium as 20, and the rhombic dodecahedron as six: beautiful whole rational numbers. 461.05 The icosahedron goes out of rational tunability due to its radius being too little to permit it having the same-size nuclear sphere, therefore putting it in a different frequency system. So when we get into atoms, we are dealing in each atom having its unique frequencies. 461.06 In the symmetrical jitterbug contraction, the top triangle does not rotate. Its vertex always points toward the mid-edge of the opposite triangle directly below it. As the sequence progresses, the top triangle approaches the lower as a result of the system's contraction. The equator of the system twists and transforms, while the opposite triangles always approach each other rota- tionlessly. They are the polar group. 461.07 Octahedron: When the jitterbug progresses to the point where the vector edges have doubled up, we arrive at the octahedron. At this stage, the top triangle can be pumped up and down with the equatorial vectors being rotated first one way and then the other. There is a momentum of spin that throws a twist into the system-positive and negative. The right-hand octahe- dron and the left-hand octahedron are not the same: if we were to color the vectors to identify them, you would see that there are really two different octahedra. 461.08 Tetrahedron: As thetop triangle still plunges toward the op- posite triangle, the two comers, by inertia, simply fold up. It has become the tetrahedron. In the octahedron stage, the vectors were doubled up, but now they have all become fourfold, or quadrivalent. The eight tetrahedra of the original vector equilibrium are now all composited as one. They could not es- cape from each other. We started off with one energy action in the system, but we have gone from a volume of 20 to a volume of one.* The finite clo- * In vectorial geometry, you have to watch for the times when things double up. The vectors represent a mass and a velocity. Sometimes they double up so they represent twice the value-or four times the value-when they become congruent. Fig. 461.08 Jitterbug System Collapses into Tetrahedron: Polarization: The "jitter- bug" system, after reaching the octahedron phase, may be collapsed and folded into the regular tetrahedron. Note that because the vector equilibrium has 24 edges the tetrahedra have accumulated four edges at each of their six normal edges. The "jitter- bug" can also be folded into a larger but incomplete tetrahedron. Note that in this case the two sets of double edges which suggest polarization.

fazer um eletroiman

rica casinha huehe

Brother Steven

Sempre fui fã do Steven Seagal aqui está porque!

Sachiko Kodama "Morpho Towers -- Two Standing Spirals" (2007)

Sachiko Kodama "Morpho Towers -- Two Standing Spirals" (2007) Collaborator: Yasushi Miyajima (Sony CSL) Special Thanks:Satoru Saito, Kingo Arakawa, Takeshi Aoki, Osamu Sumiya(UEC), Megumi Sato Music: Tetsuhide Hidaka, PIRAMI Image copyright (c)Sachiko Kodama, music by Tetsuhide Hidaka (Windows Media Player) “Morpho Towers--Two Standing Spirals” is an installation that consists of two ferrofluid sculptures that moves synthetically to music. The two spiral towers stand on a large plate that hold ferrofluid. When the music starts, the magnetic field around the tower is strengthened. Spikes of ferrofluid are born from the bottom plate and move up, trembling and rotating around the edge of the iron spiral. The body of the tower was made by a new technique called “ferrofluid sculpture” that enables artists to create dynamic sculptures with fluid materials. This technique uses one electromagnet, and its iron core is extended and sculpted. The ferrofluid covers the sculpted surface of a three-dimensional iron shape that was made on an electronic NC lathe. The movement of the spikes in the fluid is controlled dynamically on the surface by adjusting the power of the electromagnet. The shape of the iron body is designed as helical so that the fluid can move to the top of the helical tower when the magnetic field is strong enough. The surface of the tower responds dynamically to its magnetic environment. When there is no magnetic field, the tower appears to be a simple spiral shape. But when the magnetic field around the tower is strengthened, spikes of ferrofluid are born; at the same time, the tower’s surface dynamically morphs into a variety of textures ranging from soft fluid to minute moss, or to spiky shark’s teeth, or again to a hard iron surface. The ferrofluid, with its smooth, black surface that seems to draw people in, reaches the top of the tower, spreading like a fractal, defying gravity. The spikes of ferrofluid are made to rotate around the edge of the spiral cone, becoming large or small depending on the strength of the magnetic field. In this work the speed of this rotation can be controlled without motors or shaft mechanisms ? we simply control the magnetic power. In this work, we are trying to activate analogue physical phenomena (= fluid) precisely by utilizing digital music metadata. To control the synchronization of the ferrofluid with the music playback in real time, time series metadata are added to the music beforehand. The metadata consist of musical information, such as beat position, chord progression, and melody block information, and ferrofluid control information such as DC bias voltage and AC pattern. Each data record has a time stamp that indicates the timing of presentation. All data are stored in time-series order. These time series metadata must be accurate for precise control of timing, so as to cancel the time delay of fluid movement. By this correction, the time when the protuberance of the spike reaches its maximum size is coincident with the beat of the music. As a result, the rhythm of the fluid movement coincides with the musical rhythm. When there is no sound, the fluid falls down into the plate. As there are two towers in the installation, complicated expressions of surfaces become possible. Each tower’s surface pulsates, like one creature calling to the other. Fluid moves synthetically with the music, as if it breathes, and the condition of the fluid's surface emerges as autonomous and complex. In this art we want to harmonize several opposing properties, such as hardness (iron) / softness (fluid) and freedom (desire for design) / restriction (natural powers such as gravity). This work emerges as an autonomous transformation of the material itself: sometimes it seems like a horn, sometimes a fir tree, and sometimes even like the Tower of Babel. -home

Alternador e Dínamo

Alternador e Dínamo

O esquema representa um gerador de corrente alternada, denominadoalternador. O conjunto de espiras é chamado armadura e seus terminais são coletados a anéis metálicos. Em cada anel apóia-se uma escova, geralmente de grafite; a corrente é entregue ao circuito através dessas escovas. Denomina-se coletor o conjunto formado pelos anéis e escovas.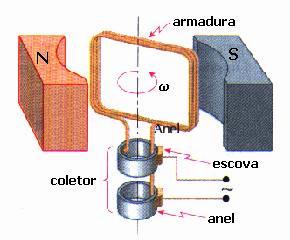

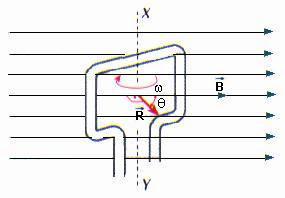	A espira girando em campo magnético uniforme, com velocidade angular constante gera uma corrente alternadainduzida.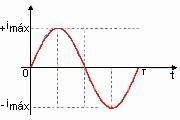

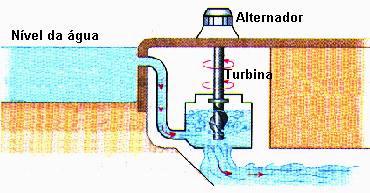	Numa usina hidrelétrica, a rotação da armadura é originada pela energia mecânica de uma turbina. Essa energia pode ser obtida através da energia potencial do desnível de uma queda d'água ou através da energia de uma máquina a vapor.

Um grande avanço tecnológico foi obtido na construção de muitos acessórios que aperfeiçoaram o funcionamento dos alternadores. Chegou-se, então, aos enormes geradores das grandes centrais elétricas, que possibilitaram a utilização da energia elétrica em larga escala. Por mais complicados que sejam esses geradores, seu funcionamento baseia-se no alternador.

Neste alternador, a substituição do par de anéis por um comutador é um artifício simples, que permite manter a corrente em um mesmo sentido. O comutador é um anel metálico dividido em dois setores, cada um ligado aos terminais da armadura. Em cada meia-volta da armadura, o comutador troca o terminal ligado ao circuito externo. Isso origina uma corrente de mesmo sentido, apesar de variar de intensidade. Tal corrente é denominada corrente pulsante. Aumentando-se o número de setores do comutador, o que é possível através do aumento do número de armaduras, obtemos uma corrente praticamente contínua no circuito extreno. Dizemos que a corrente está retificada e o aparelho constitui um dínamo.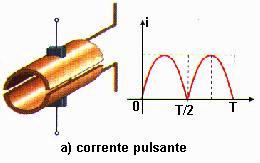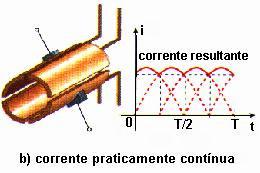

Dínamos

Recebe o nome de dínamo (da palavra grega dynamis, que significa força) um gerador de eletricidade, destinado a transformar energia mecânica em energia elétrica.
Este equipamento consiste basicamente de um ímã fixo em um eixo móvel, sendo que ao redor deste há uma bobina(um extenso fio enrolado em espiras e feito de material condutor elétrico, geralmente cobre), sem que haja contato físico entre a bobina e o ímã.
Dois tipos de dínamo são utilizados:

• dínamo em corrente contínua, no qual seu conjunto fornece corrente contínua, ou seja, corrente que circula num só sentido apenas.
• o alternador, ou dínamo de corrente alternada, fornecedor de corrente alternada, corrente que circula em um e outro sentido alternadamente. Há certa confusão entre estas denominações, e algumas fontes costumam-se referir a este conjunto especificamente como alternador, apenas. Assim, dínamo seria somente o gerador de corrente contínua.

O princípio de funcionamento de um dínamo está ligado ao fenômeno da indução eletromagnética num quadro plano que gira em campo magnético unifome. Tal fenômeno pode ser explicado pela Lei de Lenz, que estabelece que o sentido da corrente induzida é oposto da variação do campo magnético que a gera. Assim, a variação de um campo eletromagnético gera corrente elétrica. Tanto no dínamo de corrente alternada como no de corrente contínua o quadro é percorrido por corrente alternada. A diferença entre eles está na maneira de colher essa corrente para fora do quadro. Essa captação da corrente para fora do quadro é feita por um dispositivo chamado coletor.
O desenvolvimento do dínamo inicia-se com as pesquisas de Hans Orsted, que, ao observar que a agulha de umabússola oscilava quando aproximada a um fio condutor percorrido por corrente elétrica. Posteriormente, Michel Faraday se interessou pelo fenômeno e após alguns experimentos, observou que quando um imã se move próximo de um circuito elétrico ativo, a corrente elétrica do circuito é alterada.
Os principais componentes de um dínamo são:

• campo eletromagnético -  Eletroímã fixo constituído por uma barra de ferro e bobinas; quando exposta a uma corrente carregada eletricamente, cria-se um campo magnético.
• comutador - Placas condutoras que são isoladas umas das outras e ligadas à bobina do campo, recebendo e retificando a corrente alternada induzida.
• eixo - Parte cilíndrica que transmite um movimento de rotação a armadura do Dínamo.
• quadro - Caixa metálica que abriga o campo magnético.
• ventoinha - Dispositivo com pás que permite a circulação do ar de modo a resfriar o dínamo.
• armadura - Parte móvel do dínamo que é constituída por uma bobina, que produz uma corrente eléctrica à medida que gira dentro do campo.
• bobina - Fio condutor que é enrolado em torno do cilindro da armadura, que gira no campo magnético produzido pelo indutor para criar uma corrente eléctrica.
• escova - Condutor que é friccionado contra as placas do comutador e transmite a corrente contínua produzida pelo dínamo a um circuito exterior.

Bibliografia:
ESTEVÃO, Vanks. Como funciona um Dínamo? Disponível em: <http://www.efeitojoule.com/2008/06/como-funciona-dinamo-bicicleta.html>. Acesso em: 25 abr. 2012.
Dínamos ou geradores mecânicos de eletricidade. Disponível em: <http://efisica.if.usp.br/eletricidade/basico/inducao/dinamos/>. Acesso em: 25 abr. 2012.
Dynamo (em inglês). Disponível em:
<http://visual.merriam-webster.com/science/physics-electricity-magnetism/generators/dynamo.php#brush31913>. Acesso em: 25 abr. 2012.

Dínamos ou geradores mecânicos de eletricidade

Chamam-se geradores mecânicos de corrente elétrica, ou dínamos, aos dispositivos que transformam energia mecânica em energia elétrica. Os dínamos se dividem em dois grandes grupos: 1º - Dínamo com corrente contínua É aquele que fornece corrente contínua, isto é, corrente que circula num único sentido. 2º - Dínamo com corrente alternada Também chamado alternador – é aquele que fornece corrente alternada, isto é, corrente que circula num sentido e noutro alternadamente. Princípio de funcionamento Os dínamos funcionam por meio de indução eletromagnética. E esta é sem dúvida a mais importante aplicação do fenômeno de indução. Isso porque esse fenômeno é o único que fornece corrente elétrica com grande energia, como por exemplo, essa corrente que é fornecida para iluminação das cidades e para as indústrias. O princípio de funcionamento dos dínamos em geral, tanto de corrente contínua como de corrente alternada, é a indução eletromagnética num quadro plano que gira num campo magnético unifome. A indução nesse quadro foi estudada no tópico "Indução num Quadro Plano em Rotação num Campo Uniforme". Vimos que, à medida que o quadro gira, ele é percorrido por uma corrente alternada senoidal . Tanto no dínamo de corrente alternada como no de corrente contínua o quadro é percorrido por corrente alternada. A diferença entre eles está na maneira de colher essa corrente para fora do quadro. Essa captação da corrente para fora do quadro é feita por um dispositivo chamado coletor. a. Coletor de corrente alternada Suponhamos que o quadro seja aberto em A e D, e daí sejam tirados dois condutores AB e CD que são ligados a dois anéis, B e D. Encostados a esses anéis existem dois pedaços de carvão (que é condutor), m e n (fig. 322). O circuito externo, R, onde vai ser utilizada a corrente, é ligado a esses pedaços de carvão. À medida que o quadro gira dentro do campo magnético, os anéis giram juntos com ele. Os pedaços de carvão m e n ficam fixos e os anéis ficam raspando neles. A corrente que se produz no quadro passa para os anéis, deste para o carvão e do carvão vai para o circuito externo. É fácil ver que, com esse dispositivo, quando a corrente elétrica muda de sentido no quadro, também muda de sentido no circuito externo, isto é, no circuito externo ela é captada também como alternada. A figura 323 mostra o quadro entre os polos do ímã que produz o campo magnético. Figura 322 Figura 323 Coletor é o nome que se dá ao conjunto dos anéis e pedaços de carvão (fig. 323). Os pedaços de carvão são chamados escovas. b. Coletor de corrente continua Em vez de dois anéis ele se compõe de dois semi-anéis. Cada semi-anel é ligado a uma ponta do quadro girando junto com o quadro. Os pedaços de carvão são fixos, e são ligados ao circuito externo. Quando os semi-anéis giram eles ficam apertados contra os pedaços de carvão (figura acima). Vemos que, em cada meio período, um semi-anel está em contato com um pedaço de carvão diferente. A posição dos carvões é ajustada de tal modo que, no instante em que a corrente muda de sentido no quadro, há troca de contato entre os carvões e os semi-anéis. Desse modo, fora do quadro, a corrente caminha sempre no mesmo sentido, isto é, é contínua.

Stop Monsanto

Dia 25 de Maio há uma marcha mundial contra a Monsanto.

Em Lisboa a marcha inicia-se no Largo Camões.
Facebook event

A Companhia Monsanto é uma indústria multinacional de agricultura e biotecnologia. Situada nos Estados Unidos, é hoje, em sua maior parte, francesa. É a líder mundial na produção do herbicida glifosato, vendido sob a marca Roundup. Também é, de longe, o produtor líder de sementes geneticamente modificadas (transgênicos), respondendo por 70% a 100% do market share para variadas culturas. Fonte: Wikipédia 

Com 110 anos de história (1901-2013), a Monsanto é a maior produtora de produtos agrícolas transgénicos do mundo.

A Monsanto é acusada de ocultar factos de agências governamentais, falsas informações nos rótulos dos seus produtos, derrames de químicos e deposição química inadequada. Encontra-se fortemente envolvida na indústria de guerra biológica e desenvolvimento de armas químicas. Tem uma história longa e suja, com responsabilidade por alguns dos compostos mais tóxicos que a humanidade conhece. Do DDT ao agente laranja e mais recentemente o "Roundup", temos muitas razões para questionar os motivos desta corporação.

Recentemente, o o Presidente e Congresso dos EUA passaram colectivamente a apelida "Lei de Protecção Monsanto" que, entre outras coisas, proíbe os tribunais de travar a venda de sementes geneticamente modificadas da Monsanto. Nos Estados Unidos, a FDA, a agência encarregada de garantir a segurança alimentar para a população, é dirigida por antigos executivos da Monsanto. Verifica-se um conflito questionável de interesses e explica a falta de pesquisa do governo para os efeitos a longo prazo dos produtos transgénicos.

Estudos têm mostrado que os alimentos geneticamente modificados da Monsanto podem levar a condições graves de saúde, como o desenvolvimento de tumores cancerígenos, infertilidade e malformações congénitas. Cientistas indicam os OGM como umas das causas do colapso mundial da população de abelhas.

Lucrou 2 bilhões de dólares em 2009, os seus lucros caíram para apenas 1 bilhão de dólares em 2010 depois de expostos alguns dos seus actos escrupulosos. Contudo a Monsanto contínua a forjar o seu monopólio sobre a oferta de alimentos do mundo, incluindo os direitos exclusivos sobre patentes de sementes e composição genética.

Sobre transgénicos e a Monsando

Operação da Monsanto na Índia
Um país com mais de 550 milhões de agricultores, em grande parte pobres, com um mercado agrícola atormentado por práticas ineficientes.

No passado, o governo indiano tentou a sua reforma através da implementação de subsídios e empréstimos aos agricultores. Por pressão do Banco Mundial e do Fundo Monetário Internacional (FMI), o governo indiano decidiu proceder a "liberalização do mercado" o que significou a remoção de todos os subsídios e empréstimos garantidos pelo Estado aos agricultores.

Nestas condições os gigantes do agro negócio entraram no mercado indiano. Houve um acordo com os governos estaduais para introduzir um Memorando de Entendimento que ditou os termos de disseminação de organismos geneticamente modificados (OGM) no mercado local.

Para a Monsanto, foi uma questão de convencer os agricultores a usar sementes geneticamente modificadas, prometendo transformar a vida dos agricultores com as suas sementes "mágicas". A empresa vendeu as suas sementes aos agricultores com esperanças de colheitas abundantes. Para muitos destes homens e mulheres, a maioria gente simples e sem muita educação, os OGM's foram vendidos como uma fórmula mágica que mudaria as suas vidas.A ironia é que as sementes transgénicas não têm sido eficazes na Índia.
Um dos problemas normais a agricultura na região é o baixo índice de pluviosidade. Os agricultores não estavam cientes de que as sementes geneticamente modificadas precisam de mais água do que as sementes tradicionais.
As sementes caras amontoaram dívidas e destruíram terras agrícolas. Em muitos casos, as culturas não se concretizaram e impossibilitaram a cultura de sementes normais nas épocas seguintes.

Sem colheitas, os agricultores não podiam pagar os empréstimos adquiridos. Sem colheitas os agricultores ficaram sim sementes para plantar a próxima colheita, sendo obrigados a comprar mais sementes.

Sobrecarregados por dívidas, muitos acabaram com as suas próprias vidas, alguns até mesmo pela ingestão dos pesticidas tóxico Roundup, produto da Monsanto. Até o momento, cerca de 150 mil agricultores acabaram com as suas vidas no que é popularmente chamado de "genocídio GM". Muitas famílias são forçadas a herdar dívida por medo de perder suas casas e terras.



Mais informação:
http://www.organicconsumers.org/
Top 10 Facts YOU Should Know About Monsanto
Lethality of Roundup 'Weedkiller' Extends Beyond Plants To Humans
Miracle grow Indian farmers smash crop yield records without GMOs.